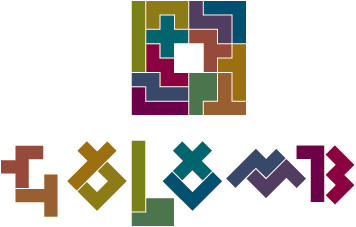
For Solomon Golomb, mathematician and inventor of pentaminoes. Created for a presentation to MathCounts, a national junior high school mathematics competition, May 10, 1996.
If two squares side by side is a "domino", then n squares joined side by side to make a shape is a "polyomino", an idea invented by mathematician Solomon Golomb of USC. There are two distinct "trominoes" (three squares): a straight line and an L. There are five distinct "tetraminoes" (four squares), popularized in the computer game Tetris, which was inspired by Golomb's polyominoes.
Shown above are the twelve distinct pentaminoes -- shapes made of five squares. There are dozens of games you can play with pentaminoes, like trying to arrange them into a 5 by 12 rectangle, 6 by 10 rectangle, or 3 by 4 by 5 solid. A two-person pentamino game was filmed for the movie 2001, but was cut in favor of chess. 2001 author Arthur C. Clarke later incorporated pentaminoes in his novel The Fountains of Paradise.
If you are interested in purchasing a set of pentaminoes to play with, check out the online puzzle store Puzzletts. My favorite pentamino sets are ones made of 3-d cubes, not just flat squares, since they can be stacked into three-dimensional shapes as well as flat shapes.
There are twelve pentaminoes and six letters in "Golomb", which leads to the nice challenge of spelling "Golomb" using just two letters to make each letter shape. First I worked on the "O"s. I wanted both shapes to be the same, and to have at least mirror symmetry since they couldn't both have rectangular or square symmetry. The long piece obviously belonged with the "L", and the zig-zag piece with the "B". Making a convincing "M" was rather difficult. Finally I used the remaining pieces to make a "G", probably the weakest letter.
Solomon Golomb is a prolific inventor of interesting bits of recreational mathematics, including Rep-tiles (shapes that can be dissected into several smaller copies of the original shape) and Golomb's Ruler (if a ruler has markings only at 1, 3, 6 and 7 inches, it can still measure every integer distance from 1 to 6 inches in length). You can read more about polyominoes in Golomb's book Polyominoes, published by Princeton University Press.